Consider the decimal expansion of the reciprocal of the number seven,
 |
(1)
|
which is a repeating decimal. Now take overlapping pairs of these digits, giving (1, 4), (4, 2), (2, 
, (8, 5), (5, 7) and (7, 1).
Five points determine a conic equation. Surprisingly, all six of these points lie on the ellipse (Wells 1986)
 |
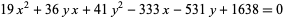(2)
|
illustrated above.
Even more surprisingly, overlapping pairs of pairs of digits, given by (14, 28), (42, 85), (28, 57), (85, 71), (57, 14), (71, 42), also give an ellipse. This ellipse has equation
 |
(3)
|
and is illustrated above.
, (8, 5), (5, 7) and (7, 1).
