The Chebotarev density theorem is a complicated theorem in algebraic number theory which yields an asymptotic formula for the density of prime ideals of a number field 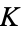 that split in a certain way in an algebraic extension
that split in a certain way in an algebraic extension 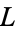 of
of 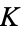 . When the base field is the field
. When the base field is the field 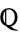 of rational numbers, the theorem becomes much simpler.
of rational numbers, the theorem becomes much simpler.
Let 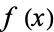 be a monic irreducible polynomial of degree
be a monic irreducible polynomial of degree 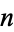 with integer coefficients with root
with integer coefficients with root 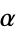 , let
, let  , let
, let  be the normal closure of
be the normal closure of 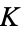 , and let
, and let 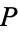 be a partition
be a partition 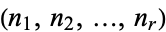 of
of  , i.e., an ordered set of positive integers
, i.e., an ordered set of positive integers 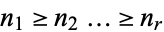 with
with  . A prime is said to be unramified (over the number field
. A prime is said to be unramified (over the number field 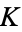 ) if it does not divide the discriminant of
) if it does not divide the discriminant of 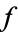 . Let
. Let 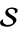 denote the set of unramified primes. Consider the set
denote the set of unramified primes. Consider the set  of unramified primes for which
of unramified primes for which  factors as
factors as 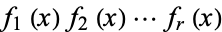 modulo
modulo 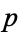 , where
, where 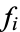 is irreducible modulo
is irreducible modulo 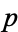 and has degree
and has degree 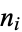 . Also define the density
. Also define the density 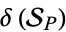 of primes in
of primes in  as follows:
as follows:
Now consider the Galois group 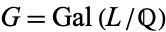 of the number field
of the number field 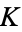 . Since this is a subgroup of the symmetric group
. Since this is a subgroup of the symmetric group 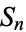 , every element of
, every element of 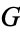 can be represented as a permutation of
can be represented as a permutation of 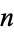 letters, which in turn has a unique representation as a product of disjoint cycles. Now consider the set of elements
letters, which in turn has a unique representation as a product of disjoint cycles. Now consider the set of elements 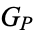 of
of 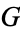 consisting of disjoint cycles of length
consisting of disjoint cycles of length 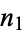 ,
, 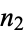 , ...,
, ..., 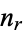 . Then
. Then 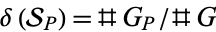 .
.
As an example, let 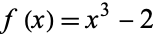 , so
, so  and
and  , where
, where 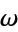 is a primitive root of unity. Since
is a primitive root of unity. Since 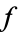 has discriminant
has discriminant 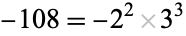 , the only ramified primes are 2 and 3.
, the only ramified primes are 2 and 3.
Let 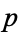 be an unramified prime. Then
be an unramified prime. Then 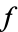 has a root (mod
has a root (mod  ) if and only if 2 has a cube root (mod
) if and only if 2 has a cube root (mod  ), which occurs whenever
), which occurs whenever  (mod 3) or
(mod 3) or  (mod 3) and 2 has multiplicative order modulo
(mod 3) and 2 has multiplicative order modulo 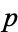 dividing
dividing  . The first case occurs for half of all unramified primes and the second case occurs for one sixth of all primes. In the first case, 2 has a unique cube root modulo
. The first case occurs for half of all unramified primes and the second case occurs for one sixth of all primes. In the first case, 2 has a unique cube root modulo 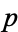 , so
, so 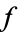 factors as the product of a linear and an irreducible quadratic factor mod
factors as the product of a linear and an irreducible quadratic factor mod 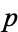 . In the second case, 2 has three distinct cube roots mod
. In the second case, 2 has three distinct cube roots mod 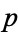 , so
, so  has three linear factors mod
has three linear factors mod 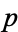 . In the remaining case, which occurs for 1/3 of all unramified primes,
. In the remaining case, which occurs for 1/3 of all unramified primes, 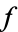 is irreducible mod
is irreducible mod 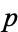 . Now consider the corresponding elements of
. Now consider the corresponding elements of  . The first case corresponds to products of 2-cycles and 1-cycles (the identity), of which there are three, or half of the elements of
. The first case corresponds to products of 2-cycles and 1-cycles (the identity), of which there are three, or half of the elements of 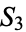 , the second case corresponds to products of three 1-cycles, or the identity, of which there is just one element, or one sixth of the elements of
, the second case corresponds to products of three 1-cycles, or the identity, of which there is just one element, or one sixth of the elements of  , and the remaining case corresponds to 3-cycles, of which there are two, or one third the elements of
, and the remaining case corresponds to 3-cycles, of which there are two, or one third the elements of 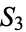 . Since
. Since  in this case, the Chebotarev density theorem holds for this example.
in this case, the Chebotarev density theorem holds for this example.
The Chebotarev density theorem can often be used to determine the Galois group of a given irreducible polynomial 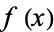 of degree
of degree 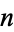 . To do so, count the number of unramified primes up to a specified bound for which
. To do so, count the number of unramified primes up to a specified bound for which 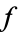 factors in a certain way and then compare the results with the fractions of elements of each of the transitive subgroups of
factors in a certain way and then compare the results with the fractions of elements of each of the transitive subgroups of 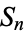 with the same cyclic structure. Lenstra provides some good examples of this procedure.
with the same cyclic structure. Lenstra provides some good examples of this procedure.