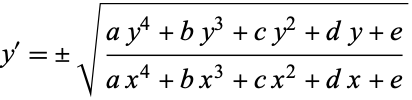AKADEMİK MATEMATİK - Euler Differential Equation

The general nonhomogeneous differential equation is given by

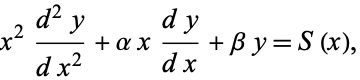(1)

and the homogeneous equation is

(2)

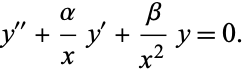(3)

Now attempt to convert the equation from

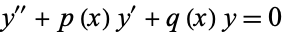(4)

to one with constant coefficients

(5)

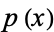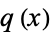by using the standard transformation for linear second-order ordinary differential equations. Comparing (3) and (5), the functions and are

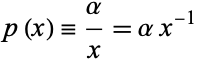(6)

(7)

Let 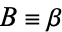and define

Then 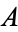is given by

which is a constant. Therefore, the equation becomes a second-order ordinary differential equation with constant coefficients

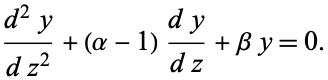(15)

Define

and

			(20)
			(21)

The solutions are

$y={c_1e^(r_1z)+c_2e^(r_2z) (alpha-1)^2>4beta; (c_1+c_2z)e^(az) (alpha-1)^2=4beta; e^(az)[c_1cos(bz)+c_2sin(bz)] (alpha-1)^2<4beta.$

(22)

In terms of the original variable ,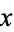

$y={c_1|x|^(r_1)+c_2|x|^(r_2) (alpha-1)^2>4beta; (c_1+c_2ln|x|)|x|^a (alpha-1)^2=4beta; |x|^a[c_1cos(bln|x|)+c_2sin(bln|x|)] (alpha-1)^2<4beta.$

(23)

Zwillinger (1997, p. 120) gives two other types of equations known as Euler differential equations,

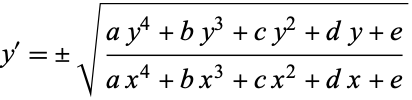(24)

(Valiron 1950, p. 201) and

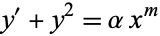(25)

(Valiron 1950, p. 212), the latter of which can be solved in terms of Bessel functions.